scipy.special.mathieu_sem

scipy.special.mathieu_sem(m, q, x, out=None) = <ufunc 'mathieu_sem'>

奇次馬 Mathieu 函數及其導數

返回 m 階和參數 q 的奇次 Mathieu 函數 se_m(x, q)，在 x 處求值（以度為單位）。同時返回 se_m(x, q) 對 x 的導數。

參數：

marray_like

函數的階數

qarray_like

函數的參數

xarray_like

函數的自變數，以度為單位，而非弧度。

outtuple of ndarray, optional

函數結果的可選輸出陣列

返回：

yscalar or ndarray

函數值

ypscalar or ndarray

對 x 的導數值

參見

mathieu_a, mathieu_b, mathieu_cem

註解

奇次 Mathieu 函數是 Mathieu 微分方程式的解

\[\frac{d^2y}{dx^2} + (b_m - 2q \cos(2x))y = 0\]

其中特徵數 \(b_m\)（使用 mathieu_b 計算）產生一個週期為 180 度（對於偶數 \(m\)）或 360 度（對於奇數 \(m\)）的奇次週期解 \(y(x)\)。

參考文獻

[1]

‘Mathieu function’。維基百科。 https://en.wikipedia.org/wiki/Mathieu_function

範例

在您的瀏覽器中試試看！

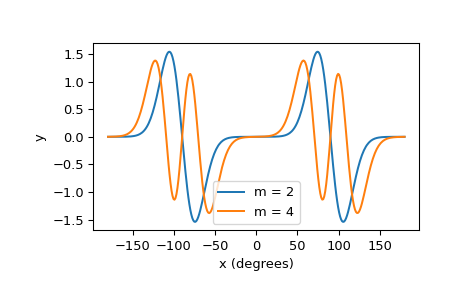
繪製 2 階和 4 階的奇次 Mathieu 函數。

>>> import numpy as np
>>> from scipy import special
>>> import matplotlib.pyplot as plt
>>> m = np.asarray([2, 4])
>>> q = 50
>>> x = np.linspace(-180, 180, 300)[:, np.newaxis]
>>> y, _ = special.mathieu_sem(m, q, x)
>>> plt.plot(x, y)
>>> plt.xlabel('x (degrees)')
>>> plt.ylabel('y')
>>> plt.legend(('m = 2', 'm = 4'))

因為 2 階和 4 階是偶數，所以每個函數的週期為 180 度。

返回在新分頁中開啟